Viscous or continuum flow
This will be found almost exclusively in the rough vacuum range. The character of this type of flow is determined by the interaction of the molecules. Consequently internal friction, the viscosity of the flowing substance, is a major factor. If vortex motion appears in the streaming process, one speaks of turbulent flow. If various layers of the flowing medium slide one over the
other, then the term laminar flow or layer flux may be applied.
Laminar flow in circular tubes with parabolic velocity distribution is known as Poiseuille flow. This special case is found frequently in vacuum technology. Viscous flow will generally be found where the molecules’ mean free path is considerably shorter than the diameter of the pipe: λ « d.
A characteristic quantity describing the viscous flow state is the dimensionless Reynolds number Re.
Re is the product of the pipe diameter, flow velocity, density and reciprocal value of the viscosity (internal friction) of the gas which is flowing. Flow is turbulent where Re > 2200, laminar where Re < 2200.
The phenomenon of choked flow may also be observed in the viscous flow situation. It plays a part when venting and evacuating a vacuum vessel and where there are leaks. Gas will always flow where there is a difference in pressure ∆p = (p1– p2) > 0. The intensity of the gas flow, i.e. the quantity of gas flowing over a period of time, rises with the pressure differential. In the case of viscous flow, however, this will be the case only until the flow velocity, which also rises, reaches the speed of sound. This is always the case at a certain pressure differential and this value may be characterized as “critical”:![∆p_(crit) = p_1[1-(P_2/P_1)_(crit)]](https://content.leybold.com/hubfs/image-Jun-25-2020-08-17-29-21-PM.png)
A further rise in ∆p > ∆pcrit would not result in any further rise in gas flow; any increase is inhibited. For air at 20,°C the gas dynamics theory reveals a critical value of
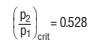
The chart below represents schematically the venting (or airing) of an evacuated container through an opening in the envelope (venting valve), allowing ambient air at p = 1000 mbar to enter. In accordance with the information given above, the resultant critical pressure is ∆pcrit = 1000 ⋅ (1– 0.528) mbar ≈ 470 mbar; i.e. where ∆p > 470 mbar the flow rate will be choked; where ∆p < 470 mbar the gas flow will decline.

Image: Schematic representation of venting an evacuated vessel
